Welcome to our comprehensive guide on equivalent expressions for the SAT Math section! Equivalent expressions are mathematical expressions that may look different but represent the same value. Understanding how to recognize and manipulate equivalent expressions is crucial for success on the SAT Math, as many questions require you to simplify, factor, or rewrite expressions. This lesson will break down the concept from the ground up, providing you with the knowledge and practice you need to tackle these problems confidently.
Mastering Equivalent Expressions for the SAT Math
Introduction
What are Equivalent Expressions?
Equivalent expressions are mathematical expressions that represent the same value, regardless of what values are substituted for the variables.
An algebraic expression consists of two or more numbers and variables combined using mathematical operations such as addition (+), subtraction (−), multiplication (×), or division (÷).
For example, and are equivalent expressions because they always yield the same result for any value of . On the SAT, you'll need to recognize when expressions are equivalent and be able to convert between different forms. This involves understanding algebraic properties like the distributive property, combining like terms, factoring, and working with exponents. Recognizing equivalent expressions allows you to simplify complex problems and find more efficient solution paths.
Free Full Length SAT Tests withOfficial-StyleQuestions
Practice with our full length adapive full testreal test-likequestions and proven300+points score boost
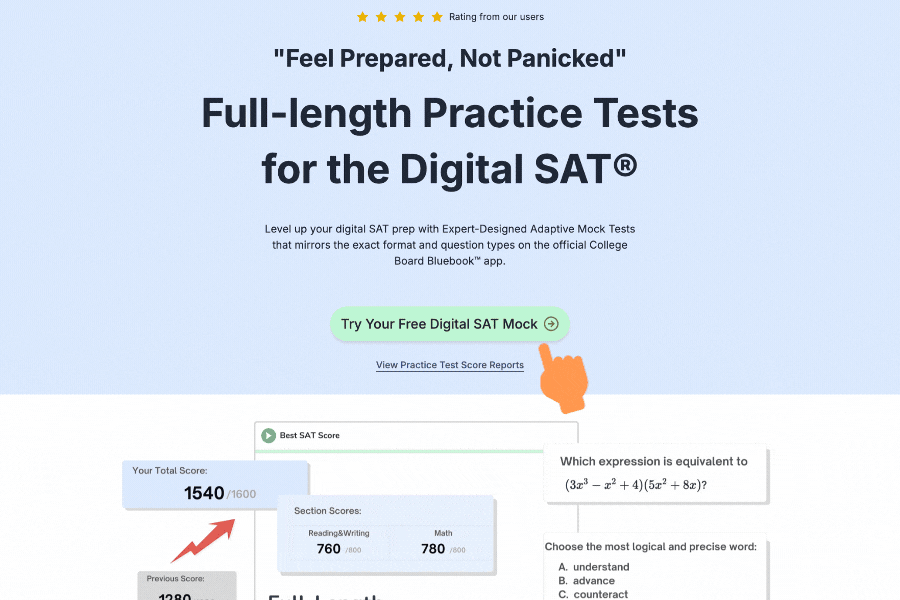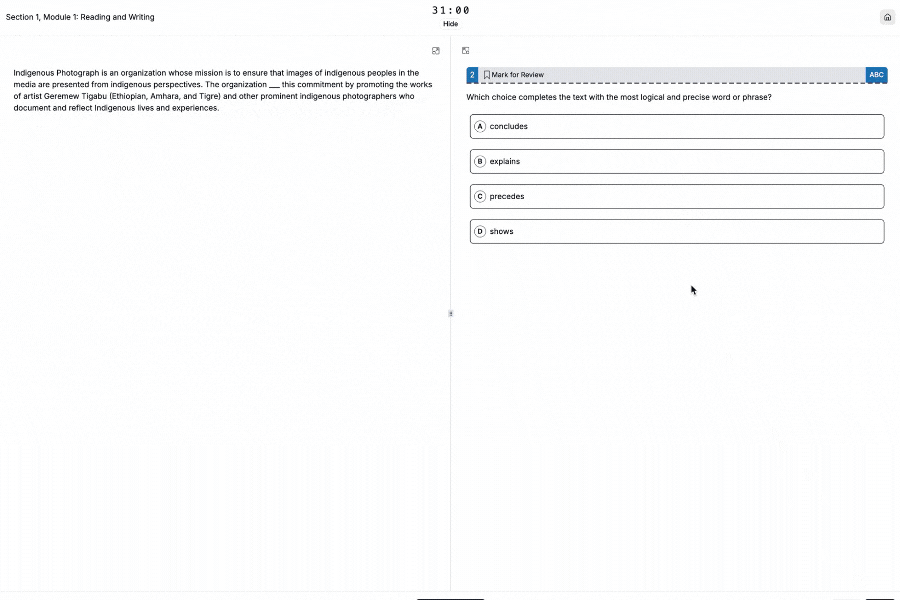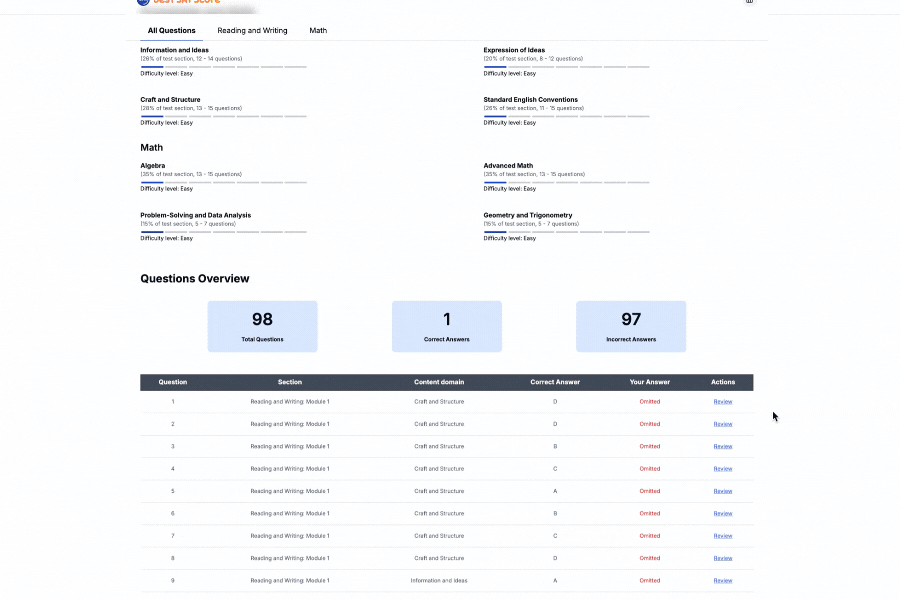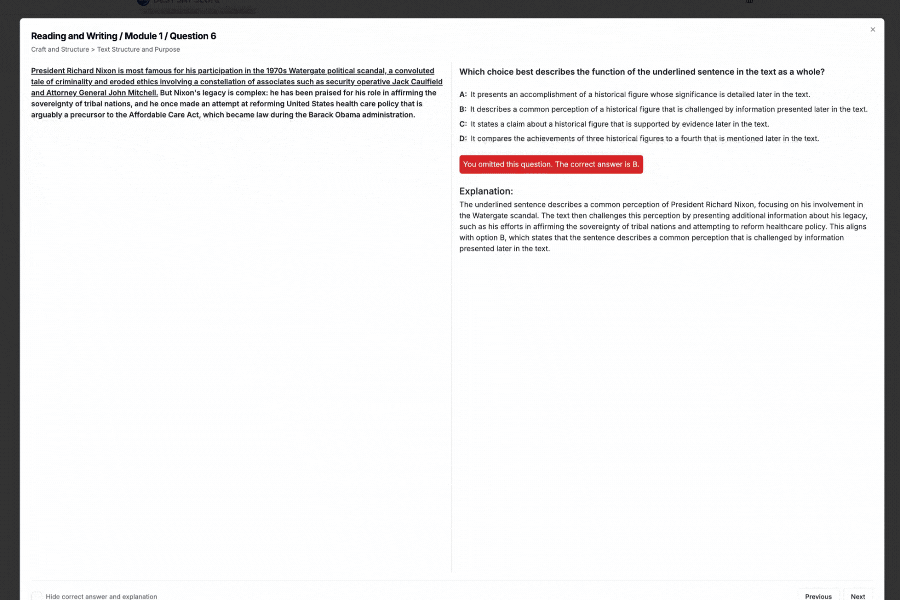
How to Use Equivalent Expressions
To effectively work with equivalent expressions on the SAT Math section, follow these key strategies:
-
Simplify expressions by combining like terms. For example, .
-
Apply the distributive property to expand expressions: . For instance, .
-
Factor expressions to find equivalent forms. For example, .
-
Recognize special patterns such as:
- Perfect square trinomials:
- Difference of squares:
- Sum of cubes:
- Difference of cubes:
-
Use exponent rules to simplify expressions with powers:
-
Substitute values to check if expressions are equivalent when you're unsure.
Equivalent Expressions Worksheet
Practice identifying and creating equivalent expressions with these exercises:
Part A: Identify whether the following pairs of expressions are equivalent.
- and
- and
- and
- and
Part B: Convert each expression to the indicated form.
- Expand:
- Factor completely:
- Simplify by combining like terms:
- Write as a single fraction:
Part C: Create an equivalent expression.
- Write an equivalent expression for in factored form.
- Write an equivalent expression for that is defined when .
Equivalent Expressions Examples
Example 1
Expanding using the distributive property
Expand the expression:
Solution:
The expressions and are equivalent.
Example 2
Factoring a quadratic expression
Factor:
Solution:
We need to find two numbers that multiply to give 12 and add to give -7.
These numbers are -3 and -4 since (-3)(-4) = 12 and (-3) + (-4) = -7.
Therefore,
The expressions and are equivalent.
Example 3
Working with perfect square trinomials
Rewrite as a perfect square:
Solution:
This is a perfect square trinomial in the form
Here, and since and
Therefore,
The expressions and are equivalent.
Example 4
Simplifying rational expressions
Simplify:
Solution:
First, factor the numerator:
Now we can cancel the common factor :
for
The expressions and are equivalent for all values of except .
Example 5
Working with exponents
Simplify:
Solution:
Using exponent rules:
Now multiply these expressions:
The expressions and are equivalent.
Example 6
Combining like terms
Simplify:
Solution:
Group like terms:
Combining these results:
The expressions and are equivalent.
Free Full Length SAT Tests withOfficial-StyleQuestions
Practice with our full length adapive full testreal test-likequestions and proven300+points score boost

Common Misconceptions
When working with equivalent expressions, students often make these mistakes:
-
Incorrect distribution: Remember that , not . For example, , not .
-
Cancellation errors: When simplifying fractions like , you cannot cancel the terms. You can only cancel factors, not terms. For example, in , you can cancel to get .
-
Exponent confusion: Remember that . The correct expansion is .
-
Factoring mistakes: Not all quadratics factor nicely. For example, cannot be factored using integer coefficients.
-
Domain restrictions: When simplifying rational expressions, always check for domain restrictions. For example, only when .
-
Square root simplification: . This is only true in special cases, like .
Practice Questions for Equivalent Expressions
Question 1
If and , which of the following statements is true?
A) for all values of
B) only when
C) only when
D) for all values of
Solution: To determine if and are equivalent, expand .
Comparing with , we see that . Since they differ by a constant, they are not equivalent for any value of . The answer is D.
Question 2
Which of the following is equivalent to for ?
A)
B)
C)
D)
Solution: To find an equivalent expression, we can use polynomial division or factoring.
Let's factor the numerator:
Now we can simplify:
for
The answer is A.
Equivalent Expressions Questions
Question 1
Which of the following expressions is equivalent to ?
A)
B)
C)
D)
Solution: Let's expand the expression step by step.
The answer is A.
Question 2
If and , which of the following is equivalent to ?
A)
B)
C)
D)
Solution: First, factor both the numerator and denominator.
These expressions cannot be simplified further since there are no common factors. The answer is A.
Equivalent Expressions Learning Checklist
I can apply the distributive property to expand expressions like .
I can combine like terms to simplify expressions.
I can factor quadratic expressions into the form .
I can recognize and work with special patterns like perfect square trinomials and difference of squares.
I can apply exponent rules correctly to simplify expressions with powers.
I can simplify rational expressions by factoring and canceling common factors.
I can identify domain restrictions when working with rational expressions.
I can verify if two expressions are equivalent by substituting values or algebraic manipulation.
Essential SAT Prep Tools
Maximize your SAT preparation with our comprehensive suite of tools designed to enhance your study experience and track your progress effectively.
Personalized Study Planner
Get a customized study schedule based on your target score, available study time, and test date.
Expert-Curated Question Bank
Access 2000+ handpicked SAT questions with detailed explanations, organized by topic and difficulty level.
Smart Flashcards
Create and study with AI-powered flashcards featuring spaced repetition for optimal retention.
Score Calculator
Convert raw scores to scaled scores instantly and track your progress towards your target score.
Topic-Focused Mini Tests
Practice specific topics with timed mini-tests to build confidence and improve accuracy.
Full-Length Practice Tests
Experience complete SAT exams under realistic conditions with adaptive difficulty.
Pro Tip
Start your SAT prep journey by creating a personalized study plan 3-4 months before your test date. Use our time management tools to master pacing, combine mini-tests for targeted practice, and gradually progress to full-length practice tests. Regular review with flashcards and consistent practice with our question bank will help you stay on track with your study goals.